-
There is variety of dominoes organization in element sets.
Traditional dominoes games present sets of dominoes elements (tiles, tokens,
printed cards), on which digits are
applied. The number of set elements is limited by number of combinations of
digit pairs from 0 to N. The most well-known set of dominoes have combination of digits from
0 to 6. Theoretically, dominoes digit interval can be from 0 to any other digit.
In addition, some digit combination can be repeated or can be absent.
The playing board can be divided into different number of squares in accordance
with number of dominoes elements. And the number of dominoes elements can be brought into
conformity with number of playing board squares. In other words,
the most optimal playing board is presented in the present dominoes game set as the playing board consisting of 8
vertical and the 8 horizontal square rows. And in accordance with above
mentioned there were presented sets of dominoes elements, which were organized so that
they can be used for playing on the available board. But in addition to it we
may calculate different ratio of dominoes elements in accordance with configuration
of different playing boards.
Dominoes digit combinations can be presented as in the following table.
-
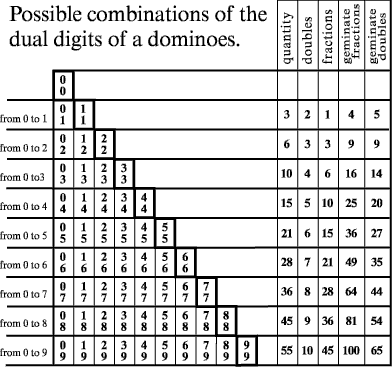 |
The lines in this table show digit interval increasing from 0 to N. In right columns there are shown number of dominoes elements in sets, which correspond to lines, and also quantities of doubles and fractions in corresponding sets. In addition numbers of dominoes elements in sets with geminated doubles and geminated fractions are presented too. It means that there are quantities of dominoes elements for the case when doubles or fractions will be presented in sets twice. Suppose that set with combination of digit from 0 to 9 includes 55 elements (decimal dominoes). Namely decimal dominoes consists of 10 doubles and 45 fractions. If this set includes geminated doubles it contains 65 dominoes elements, and if this decimal set includes geminated fractions it contains 100 dominoes elements. |
In this table there are shown only main capabilities for
changing numbers of dominoes elements. But actually these variations can be very
different.
I can carry out more detail study for some dominoes sets.
● Traditional dominoes set
(digit interval from 0 to 6) includes 28 dominoes elements. The playing board, which
consists of 8 horizontal and 7 vertical square rows, corresponds to this number
of dominoes elements.
Traditional dominoes set’s elements located on schematic squares of playing board are shown in
the Figure.
 |
Dominoes elements are distributed into proportional playing groups, which are
located on opposite board halves. Because of 14 dominoes elements of each
proportional group fully occupy squares of two limiting horizontal lines
of the playing board, which has 7 vertical and 8 horizontal rows, fits to this
number of dominoes elements. In other way, you can use the
playing board, which has 7
vertical and 7 horizontal rows. The method, by which orienting arrows are applied onto dominoes elements, is shown on the right side of the Figure. It means that this Figure is unique technical drawing, in accordance with which this set of dominoes elements can be manufactured. The digit sums, which calculated in vertical and horizontal direction, are obtained when dominoes elements are located in specific playing position, are shown as numbers situated outside the playing board borders. As can be seen from this result traditional set’s elements compose the “magic square” too, as well as the main dominoes sets, as the sum of digits on each vertical 24, and the sum of digits on each horizontal 21 or 21+21=42. Look the information on magic squares and numerological numbers on pages of other website: www.numeralgame.64g.ru/en.htm. |
-
● The dominoes set with combinations of digits from 0 to 7 and with
geminated quantity of elements with fractions.
This set exactly fits for playing board, which consist of 8
horizontal and 8 vertical square rows, because it contains 64 dominoes elements, which
corresponds to 64 squares of the playing board.
This dominoes set can be included in this game package, namely can be the fourth set
in the offered game package and to be designated by “D” letter. This
fourth set of dominoes elements
look like they are shown in the Figure.
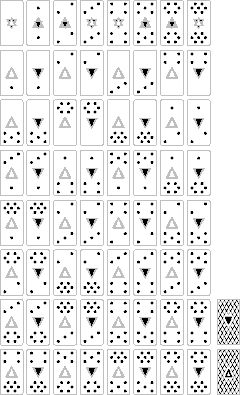 |
Dark and light triangles identify dominoes elements, which belong to two
proportional groups in the fourth set composition, and also are orienting
designations, in accordance to which dominoes elements can be oriented during
their location onto the playing board. Doubles are designated by other way because their orientation has no meaning. But doubles belong to different proportional groups too and they have corresponding designations. These dominoes elements are designated by corresponding contrast triangles on their back sides. This dominoes set can be reduced. For example,
dominoes elements including digit 7 can be deleted and all set will
include 49 elements. In this case the playing board consisting of 7 vertical and 7
horizontal square rows will be the corresponding board. |
Matrix of dominoes digits.
 |
Digits are applied on dominoes elements of the fourth set with help of
unusual method. Hexadecimal notation in place of decimal dominoes notation was
used in this case. This notation allows applying on dominoes elements digits from 0 to 15. It means that dominoes dots can have 16 meanings as the matrix shows. Due to this fact different dominoes sets may be organized. Some versions of digit presentations are shown in the Figure only. Nevertheless actual positions of dots for one or another digit can differ from proposed ones. |
Altogether, there are can be many different dominoes sets.
Many possible sets were not considered. But if results of different researches
of dominoes mathematical systems will be interesting for you they can be
presented on this website pages.